Refer to BITSAT Mathematics Continuity and Differentiability MCQs provided below available for download in Pdf. The MCQ Questions for Full Syllabus Mathematics with answers are aligned as per the latest syllabus and exam pattern suggested by BITSAT, NCERT and KVS. Continuity and Differentiability Full Syllabus MCQ are an important part of exams for Full Syllabus Mathematics and if practiced properly can help you to improve your understanding and get higher marks. Refer to more Chapter-wise MCQs for BITSAT Full Syllabus Mathematics and also download more latest study material for all subjects
MCQ for Full Syllabus Mathematics Continuity and Differentiability
Full Syllabus Mathematics students should refer to the following multiple-choice questions with answers for Continuity and Differentiability in Full Syllabus.
Continuity and Differentiability MCQ Questions Full Syllabus Mathematics with Answers
Question: The function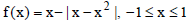 
is continuous on the interval
- a) [–1, 1]
- b) (–1, 1)
- c) {–1, 1] – { 0 }
- d) (–1, 1) – {0}
Answer: [–1, 1]
Question: If
then f(x) is
- a) Continuous as well as differentiable at x = 0
- b) Continuous but not differentiable at x = 0
- c) Differentiable but not continuous at x = 0
- d) Neither continuous nor differentiable at x = 0
Answer: Continuous as well as differentiable at x = 0
Question: If f (x) =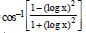
then the value of f ' (e) is equal to
- a) 1
- b)
- c)
- d)
Answer:
Question: The number of points at which the function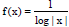 
is discontinuous is :
- a) 1
- b) 2
- c) 3
- d) 4
Answer: 3
Question: Let f (x) =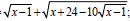 
be real valued function. Then f ¢(x) for 1 < x < 26 is
- a) 0
- b)
- c) 2 √x -1-5
- d) None of these
Answer: 0
Question: For any differentiable function y of x,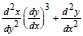 
- a) 0
- b) y
- c) – y
- d) x
Answer: 0
Question: If a function f(x) is given by
then at x = 0, f(x)
- a) Has no limit
- b) Is not continuous
- c) Is continuous but not differentiable
- d) Is differentiable
Answer: Is not continuous
Question: If g is the inverse of function f and f ¢(x) = sin x, then g¢(x) is equal to
- a) cosec {g(x)}
- b) sin {g(x)}
- c)
- d) None of these
Answer:
Question: The number of real roots of the equation ex–1 + x – 2 = 0 is
- a) 1
- b) 2
- c) 3
- d) 4
Answer: 1
Question: If y =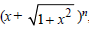
then 
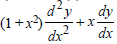is
- a) n2y
- b) – n2y
- c) –y
- d) 2x2y
Answer: n2y
Question:
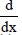(cos–1 x + sin–1 x) is
- a) π/2
- b) 0
- c)
- d) None of these
Answer: 0
Question:
Let ( ) =
Then which one of the following is true?
- a) f is differentiable at x = 0 and x =1
- b) f is differentiable at x = 0 but not at x = 1
- c) f is differentiable at x = 1 but not at x = 0
- d) f is neither differentiable at x = 0 nor at x =1
Answer: f is differentiable at x = 0 but not at x = 1
Question: If y =1 + x +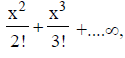
then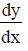
is equal to
- a) x
- b) 1
- c) y
- d) None of these
Answer: y
Question: If
is continuous at x = –5, then the value of ‘a’ will be
- a) 3/2
- b) 7/8
- c) 8/7
- d) 2/3
Answer: 7/8
Question: If x = a sin θ and y = b cos θ, then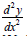 
is
- a)
- b)
- c)
- d)
Answer:
Question: If f(x) = xα log x and f(0) = 0, then the value of α for which Rolle’s theorem can be applied in [0, 1] is
- a) –2
- b) –1
- c) 0
- d) 1/2
Answer: 1/2
Question: If the function f(x) =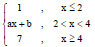
is continuous at x = 2 and 4, then the values of a and b are
- a) 3, 5
- b) 3, – 5
- c) 0, 3
- d) 0, 5
Answer: 3, – 5
Question: If y = xx2 , then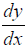 
is equal to
- a) (2 ln x)
- b) (2 ln x +1)
- c) (ln ln x +1)x x2
- d) None of these
Answer: None of these
Question: The function f (x) = (x -1) √| ln x | is at x = 1
- a) Discontinuous
- b) Continuous but not differentiable
- c) Differentiable with f ' (1) = 0
- d) Differentiable with f ¢ (1) ≠ 0
Answer: Differentiable with f ' (1) = 0
Question: Let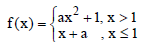 
Then f (x) is derivable at x = 1, if
- a) a = 2
- b) a = 1
- c) a = 0
- d) a = 1/2
Answer: a = 1/2
Question: If y = e–x cos x and y4 + ky = 0, where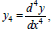 
then k =
- a) 4
- b) – 4
- c) 2
- d) – 2
Answer: 4
Question: The set of points of discontinuity of the function 1/log | x | is –
- a) {–1, 0, 1}
- b) {0}
- c) {0, 1}
- d) None of these
Answer: {–1, 0, 1}
Question: If y = (cos x2)2 then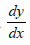 
is equal to :
- a) – 4x sin 2x2
- b) – x sin x2
- c) – 2x sin 2x2
- d) – x cos 2x2
Answer: – 2x sin 2x2
Question: If
then the points of discontinuity of the function f [ f {f(x)}] are
- a) {0, –1}
- b) {0,1}
- c) {1, –1}
- d) None of these
Answer: {0,1}
Question: If
f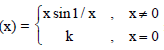
is continuous at x = 0, then the value of k will be
- a) 1
- b) -1
- c) 0
- d) None of these
Answer: 0
Question: Let
Then f (x) is derivable at x = 1, if
- a) a = 2
- b) a = 1
- c) a = 0
- d) a = 1/2
Answer: a = 1/2
Question: Let h(x) = min {x, x2}, for every real number of x, Then
- a) h is continuous for all x
- b) h is differentiable for all x
- c) h’(x) = 2, for all x > 1
- d) h is not differentiable at three values of x.
Answer: h is continuous for all x
Question: If f(x) = (x + 1)cot x is continuous at x = 0, then f(0) is equal to
- a) 0
- b) 1
- c) 1/e
- d) e
Answer: e
Question: If 2t = v2, then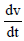 
is equal to
- a) 0
- b) 1/4
- c) 1/2
- d) 1/v
Answer: 1/v
Question: If y2 = P (x), is a polynomial of degree, then
equals
- a) P'''(x) + P' (x)
- b) P''(x). P'''(x
- c) P (x). P''' (x)
- d) a constant
Answer: P (x). P''' (x)
MCQs for Continuity and Differentiability Mathematics Full Syllabus
Expert teachers of studiestoday have referred to NCERT book for Full Syllabus Mathematics to develop the Mathematics Full Syllabus MCQs. If you download MCQs with answers for the above chapter you will get higher and better marks in Full Syllabus test and exams in the current year as you will be able to have stronger understanding of all concepts. Daily Multiple Choice Questions practice of Mathematics will help students to have stronger understanding of all concepts and also make them expert on all critical topics. After solving the questions given in the MCQs which have been developed as per latest books also refer to the NCERT solutions for Full Syllabus Mathematics. We have also provided lot of MCQ questions for Full Syllabus Mathematics so that you can solve questions relating to all topics given in each chapter. After solving these you should also refer to Full Syllabus Mathematics MCQ Test for the same chapter.
You can download the BITSAT MCQs for Full Syllabus Mathematics Continuity and Differentiability for latest session from StudiesToday.com
Yes, the MCQs issued by BITSAT for Full Syllabus Mathematics Continuity and Differentiability have been made available here for latest academic session
You can find BITSAT Full Syllabus Mathematics Continuity and Differentiability MCQs on educational websites like studiestoday.com, online tutoring platforms, and in sample question papers provided on this website.
To prepare for Continuity and Differentiability MCQs, refer to the concepts links provided by our teachers and download sample papers for free.
Yes, there are many online resources that we have provided on studiestoday.com available such as practice worksheets, question papers, and online tests for learning MCQs for Full Syllabus Mathematics Continuity and Differentiability